Mortality Risk and Cash Value Growth

On these pages, I have written several articles on the internal return on Whole Life insurance (e.g. here and here). In my examples, I often show illustrations of performance from policies that were taken out by someone in his mid-20s or 30s. 

However, for a separate project, I recently analyzed a batch of policies from various companies issued on people in their 60s, and the rates of return—even 10 years into the policy—were quite low. I quickly realized that the reason for this was that the looming death benefit played such a larger role in the valuation of these policies, versus comparable ones issued to someone at age 30.

In the present post, I’ll go through a simplistic numerical example, to show a framework for thinking about the mortality risk’s influence on the rate of growth in the cash value of a Whole Life policy.

The Example

Suppose we look at the last potential ten years of someone’s life. To keep the math easy, we assume at the start of Year 1, the person faces a 10% risk of dying that year. Then at the start of Year 2, he faces a 20% risk, at Year 3 a 30% risk, and so on. If he manages to survive to the beginning of Year 10, he faces a 100% risk of death and keels over.

Further suppose he has a fully-paid up life insurance policy, which carries a face death benefit of $1 million. (Don’t worry about dividends; assume they’re $0.) If the discount rate applied to future dollars is 5% per year, what is the actuarially fair way to appraise the value of this policy, at the beginning of each year?

The answer looks like this:

‍

‍

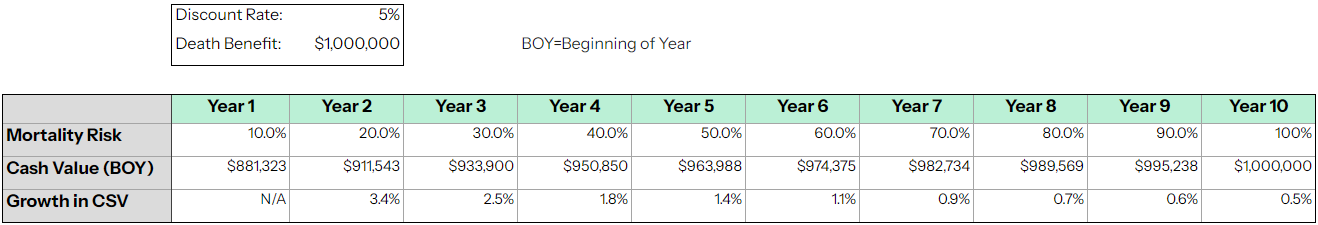
To understand the calculations in the above table, it’s easiest to start at the last year and work backwards.

At the beginning of Year 10, there is a 100% chance the person dies. So the value of the policy at that moment is clearly $1 million.

At the beginning of Year 9, there is a 90% chance the person dies—getting the immediate $1 million—and there is a 10% chance the person lives another year, to find himself holding the policy at the beginning of Year 10. We already know from the previous step that the policy is worth $1 million at the beginning of Year 10. So the risk-neutral, actuarially fair way to appraise it at the start of Year 9 is to take a probability-weighted mean of the two possible outcomes. Specifically, 90%x($1mm) + 10%x(1/1.05)x($1mm) = $995,238. Note that the discount factor is included, because at the start of Year 9, the future $1 million value of the policy (at Year 10) must be translated from future dollars to present dollars.

We’ll do just one more to establish the pattern: At the start of Year 8, there is an 80% probability of the immediate $1 million payout, and a 20% probability of getting the present discounted value of $995,238 (which is the value of the policy at the start of Year 9). So that calculation works out to a value of $989,569 at the start of Year 8.

Rates of Return

The last row of the table shows the annual growth rates of the fair market value of the policy, according to year. As the numbers indicate, the IRR on the policy starts at 3.4% from Year 1 to 2, then steadily falls down to 0.5% from Year 9 to 10. 

Superficially, this seems to confirm the warnings from critics arguing that someone with a high mortality rate will “chew up the premiums” or “cause a drag” on the growth in the policy. However, by construction, the hypothetical life insurance policy depicted in the table was priced according to fair market value and the correct actuarial procedures. Nobody would be getting “ripped off” buying into it.

Whether someone buys the policy at Year 1 or Year 9, he is still going to experience the same “average” outcome, where that term can be defined in a precise way. I’ll give some further elaboration:

At the start of Year 1, an investor would have to pony up $881,323 to buy the policy from a previous owner. (Of course we are ignoring taxes, fees, etc. in this purist analysis). When he considers the prospective performance of his new asset over the coming year, and what the possible “rates of return” on his invested principal will be, he must consider the following two outcomes:

==> There is a 90% chance he survives Year 1, and so his policy at the start of Year 2 is worth $911,543.

==> But there is also a 10% chance that he dies at the start of Year 1, in which case his estate would have $1 million that could then be put into safe bonds earning 5%. That means at the start of Year 2, in this scenario the life insurance policy his given him assets worth $1,050,000.

Now if we make the assumption that the investor is risk-neutral, then from the point of view of the investor at the start of Year 1, the policy has an “expected” value in one year’s time of 90%x$911,543 + 10%x$1,050,000 = $925,389. Compared to the original purchase price of $881,323, that represents an expected rate of return of 5%.

Now let’s look at an investor who jumped in at Year 9. He would have had to pony up a whopping $995,238 to get into the asset at that point—for a policy that only has a face value of $1,000,000. There is a 10% chance the policy grows to be $1 million, but there is a 90% chance the person dies at the start of Year 9, and the $1 million death benefit can be invested in bonds to grow to $1,050,000 by the start of Year 10. 

So again, if we assume a risk-neutral investor, at the start of Year 9 his prospective total valuation of the policy, looking forward to the possible two future “states of the world,” is 10%x$1,000,000 + 90%x$1,050,000 =  $1,045,000. Compared to the initial buy-in price of $995,238, that represents an expected 5% rate of return.

To underscore the punchline: The two italicized and bolded items show that the total expected rate of return on the asset is 5%, regardless of the mortality rate. The measured slowdown of the internal rate of return on the cash value is a subtle phenomenon, but it is a misleading indicator of the “growth” in the financial value of the policy. By construction in this example, present dollars trade at a 5% premium versus future dollars one year away.

There is a difference depending on one’s tolerance for risk. Strictly speaking, we would be on solid ground by saying that an investor buying hundreds of such policies would not make a distinction between enjoying growth due to the cash value rising, versus getting a death benefit lump sum from a mortality event.

Conclusion

The explicit internal growth rate in a life insurance policy, as expressed by the Cash Surrender Value, can be drowned out by a high mortality rate. There is some truth in the idea that a high risk of death can act as a drag on cash value growth.

However, this does NOT mean that the overall rate of financial return on such a policy would be lower, compared to a policy on a healthier (or younger) person. It’s just that with a higher mortality risk, the payout of the life insurance is itself riskier (from a financial perspective). A risk-neutral investor—such as who held hundreds of such policies in a pool—would not prefer one method of “value growth” versus the other.

‍

NOTE: This article was released 24 hours earlier on the Infinite Banking (IB) 3.0 - The Future of Finance Group

Dr. Robert P. Murphy is the Chief Economist at infineo, bridging together Whole Life insurance policies and digital blockchain-based issuance.

Twitter: @infineogroup, @BobMurphyEcon

LinkedIn: infineo group, Robert Murphy

Youtube: infineo group

To learn more about infineo, please visit the infineo website